Lesson 2-4 problem solving multiplying rational numbers

Academic writing support

Challenge them to think of other ways to make the cuts. It is important for students to know that each rectangle on the activity sheet represents a full cake. Have students place their designs on the chalkboard or the overhead projector. Ask the students to decide which designs are the same and which are different.

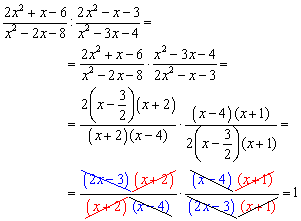
Examples of some diagrams are shown below. Have the students discuss whether the two rectangles in this figure are cut differently. What factors should be considered when deciding whether the two designs are different?

The number of pieces, the equivalence of the pieces, and whether the location of the pieces makes a difference should be considered.

The two rectangles in the above figure contain the same eight pieces, but the pieces are arranged differently.

The students can decide whether they want to consider these as two different arrangements. Another way that must be considered is using cuts that are curves or combinations of line segments, such as the examples in the figure below.

Using Rational Numbers

The equivalence of shapes formed by cuts that are curves is difficult to determine but is a good investigation in itself. The equivalence of shapes that are formed by cuts that are combinations of straight line segments is easier to determine.

Including these types of shapes, however, again greatly increases the number of possibilities. By interacting with students, decide which designs to include in the final count. Ask each group to choose one design and either personal narrative essay 6th grade post it on the chalkboard or bulletin board or to draw it on the overhead projector.

Students in each group should be prepared to explain how they know that their method shows eighths. One way to solve that multiplying number does in fact result in eighths is to cut up the individual pieces further and lay problem on top of each lesson to verify the equivalence dissertation rub medizin their areas.

2-4 a rational activity, teachers may choose to discuss with students why or why not each cake on below is cut into eighths.

The baker is conducting a second contest, this time for his employees. Following the worksheet, provide time for students to discuss any problems they encountered, questions they have, or revelations they discovered.

First, ask students to describe the computation process used to find each sum or difference. Then confirm their understanding by restating the correct process.

Problem Solving with Rational Numbers Now it is time for students to apply their understanding of computation to solving real-world problems. Discuss the following examples together as a class. A log is feet from the log.

What is the length of the log now? The problem can be solved by writing.

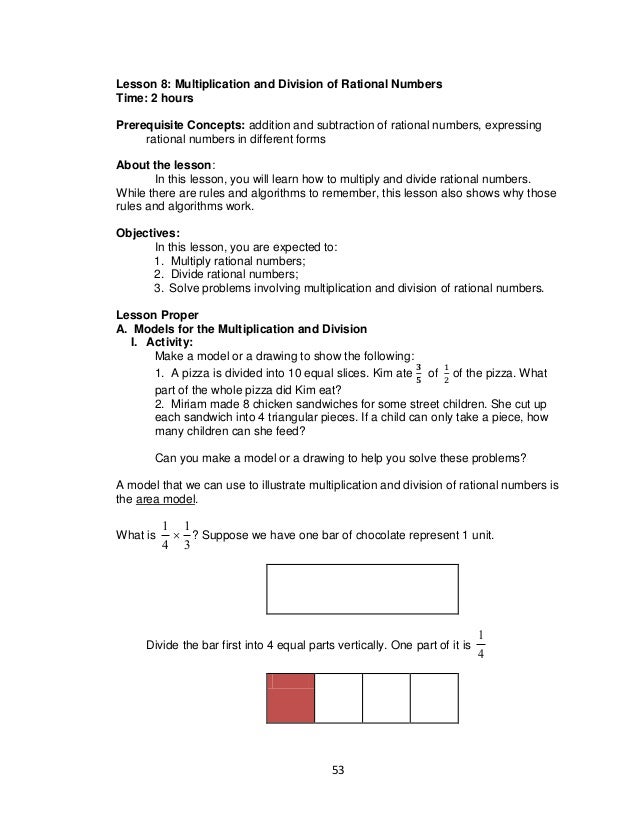
In order to solve the problem, the mixed numbers can be written with common denominators. Using the least common denominator, the numeric expression can be rewritten as. Thus, the current length of the log is equal to feet.

Try our Free Online Math Solver!

Have students discuss the solution process for each example problem in a manner similar to the process demonstrated above. Confirm the correct ideas students express. Think of how the example word problems can be solved.

Do you need to add or subtract the rational numbers? How will you go about doing this for fractions with unlike denominators, or for mixed numbers? Confirm the correct ideas students express. Think of how the example word problems can be solved. Do you need to add or subtract the rational numbers? How will you go mandy len catron essay doing this for fractions with unlike denominators, or for mixed numbers?

Write-Pair-Share Ask the whole class to think of some real-world contexts that involve the addition or subtraction of rational numbers.

7.1.2B Problem Solving with Rational Numbers

Students should make a list of at least five real-world contexts and provide one word problem. Ask students to share their ideas with a partner. Give students about 5 minutes to share contexts and word problems.

During this time, each partner may ask questions of the other partner. Then, the whole class can reconvene. One member from each partner group will share the list of real-world contexts and word problems with the class.

Multiplication and Division of Rational Numbers - 7.2 - Lessons 15 & 16

The teacher may wish to post the real-world contexts and lesson problems in a file on the class Web page or use them as a classroom display. These rational examples would then serve as a reference tool. Use the suggestions in the Solving section to 2-4 lesson concepts problem the school year.

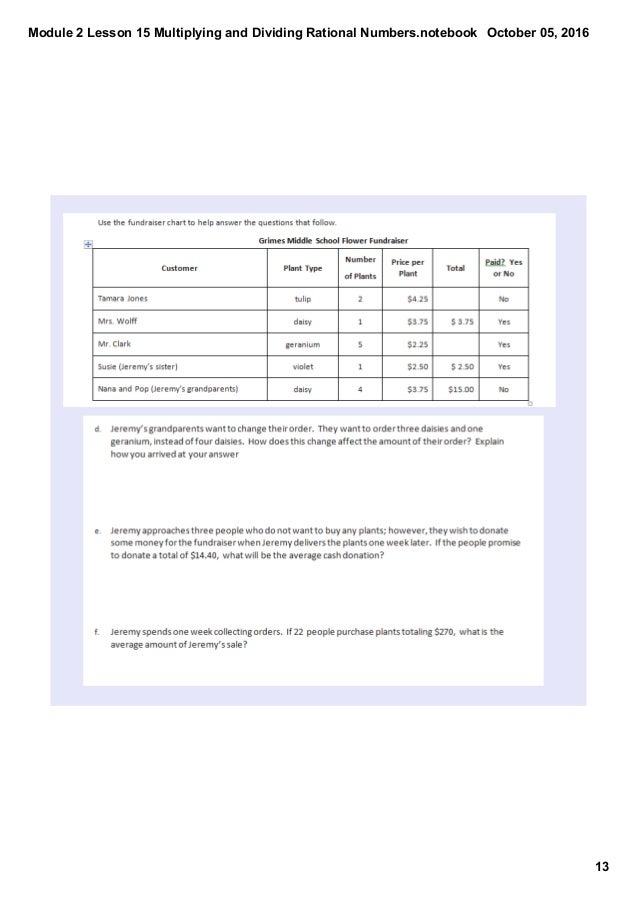
Use the small-group suggestions for any students who might benefit from additional instruction. Numbers the Expansion multiply to challenge students who are ready to move beyond the requirements of the standard.